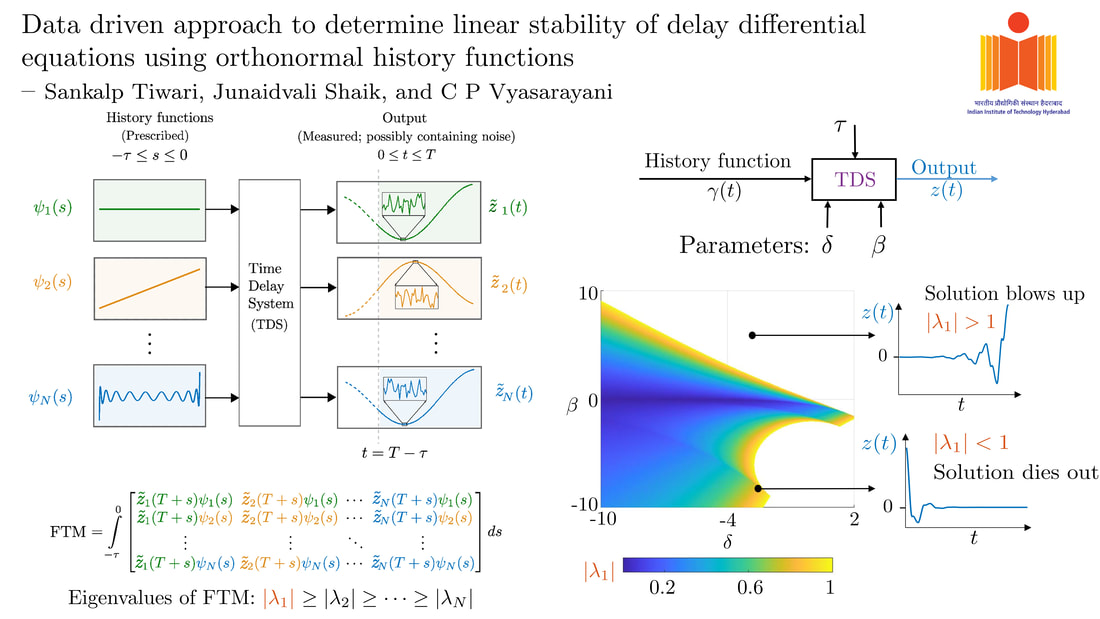
Sankalp Tiwari, Junaidvali Shaik, and C. P. Vyasarayani
J. Comput. Nonlinear Dynam. Feb 2024, 19(2): 021002 
​https://doi.org/10.1115/1.4064251

​Delayed systems are those in which the present dynamics is governed by what happened in the past. They are encountered in manufacturing, biology, population dynamics, control systems, etc. Determining stability of such systems is an important and difficult problem. In the existing works, stability is determined by assuming the governing differential equation. However, the equation may not be known or difficult to obtain. Unlike existing works, our method determines the linear stability of a delayed system using its response to a few known inputs. In particular, our method does not require or assume the differential equation governing that system. The only system information we use is its largest delay time, and the only assumption we make about the underlying equation is that its coefficients are either constant or time-periodic. Our approach involves giving the first few functions of an orthonormal polynomial basis as input and measuring/computing the corresponding responses to generate a state transition matrix, whose largest eigenvalue determines the stability. We demonstrate our method's correctness, efficacy, and convergence by studying four candidate DDEs with differing features. Importantly, we show that our approach is robust to noise in measurement, thereby establishing its suitability for practical applications.

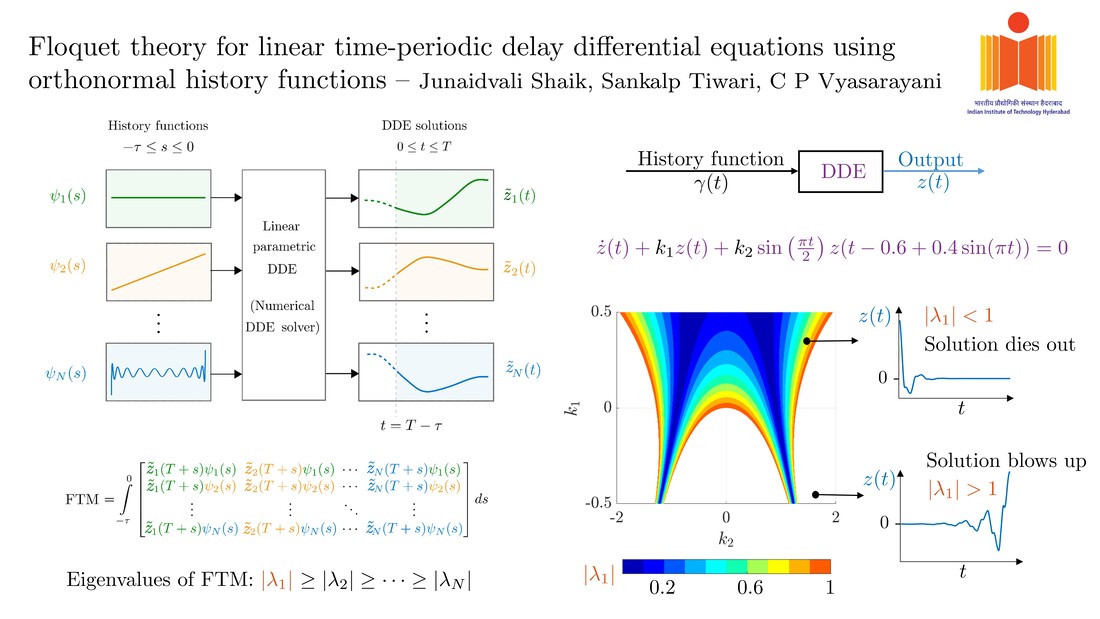
Junaidvali Shaik, Sankalp Tiwari, and C. P. Vyasarayani
J. Comput. Nonlinear Dynam. Sep 2023, 18(9): 091005, https://doi.org/10.1115/1.4062633​

Delayed systems are those in which the present dynamics is governed by what happened in the past. They are encountered in manufacturing, biology, population dynamics, control systems, etc. Determining linear stability of such systems is an important and difficult problem. In this work, we propose a new method to determine stability of time-periodic delay differential equations (DDEs). In the usual approaches, the DDE is converted into an approximate system of time-periodic ordinary differential equations (ODEs). Later, standard ODE techniques are employed. In this paper, we develop a method that is more direct and general. Our approach is analogous to the well-known Floquet theory for ODEs: we consider one polynomial basis function at a time as the input function and stack the coefficients of the corresponding DDE solutions to construct a matrix whose largest magnitude eigenvalue determines linear stability. We demonstrate the correctness, efficacy and convergence of our method by studying several candidate DDEs with time-periodic parameters and/or delays, and comparing the results with those obtained from other standard methods. Our approach has the following additional advantages: (a) it is parallelizable, (b) it converges quickly, and (c) it requires knowledge of only elementary linear algebra and numerical computation of DDE solutions. ​