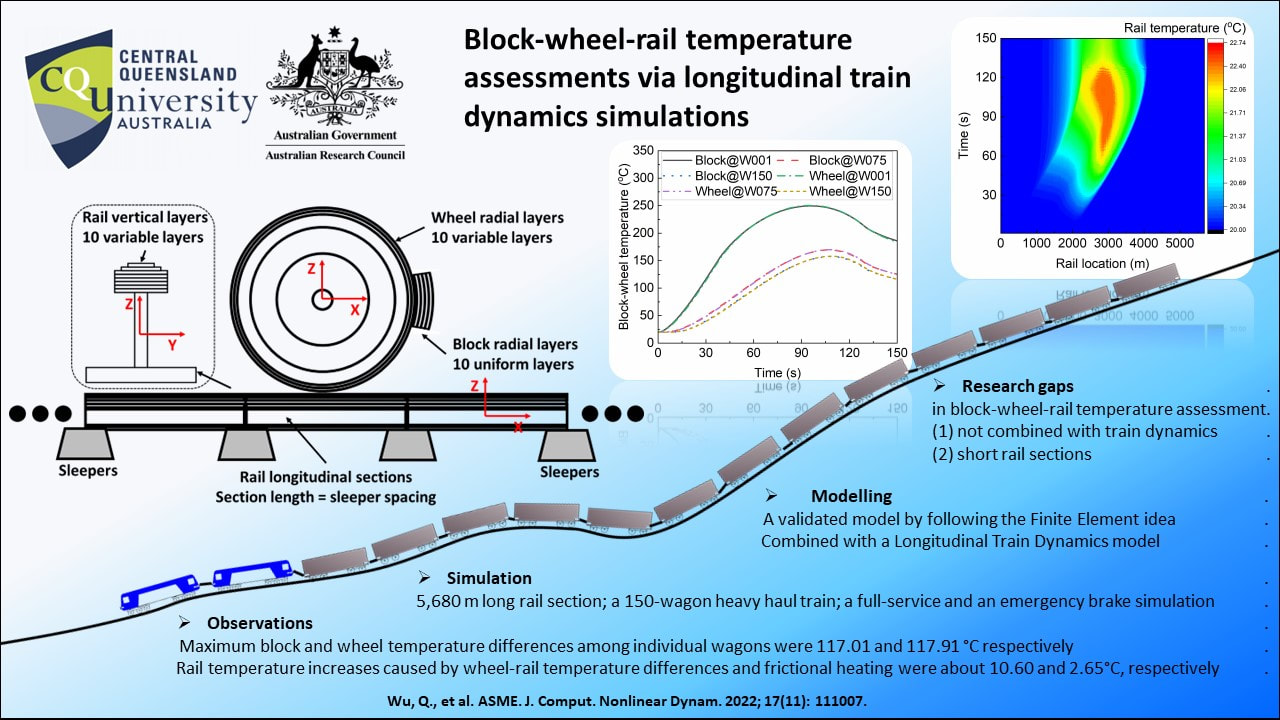
Wu, Q., Spiryagin, M., and Cole, C. (September 16, 2022). "Block–Wheel–Rail Temperature Assessments Via Longitudinal Train Dynamics Simulations." ASME. J. Comput. Nonlinear Dynam. November 2022; 17(11): 111007. https://doi.org/10.1115/1.4055431
​

​Two research gaps were identified in block-wheel-rail temperature assessment. First, current studies are not combined with train dynamics which are better descriptions of the block-wheel-rail working environment. Second, current studies cannot simulate long rail sections. This paper developed a block-wheel-rail temperature assessment model by following the Finite Element idea. Models were validated by comparing with ANSYS Finite Element models and measured data. Case studies were carried out by combining the temperature model with a Longitudinal Train Dynamics model. A full-service and an emergency brake simulation were carried out for a 150-wagon heavy haul train on a 5,680 m long rail section. The results show that, due to brake force differences at different wagon positions, the maximum block and wheel temperature differences among individual wagons in the full-service brake simulation were 117.01 and 117.91 °C respectively. This highlighted the contribution of introducing train dynamics into block-wheel-rail temperature assessment. Rail temperature increases caused by wheel-rail temperature differences and frictional heating were about 10.60 and 2.65°C, respectively.

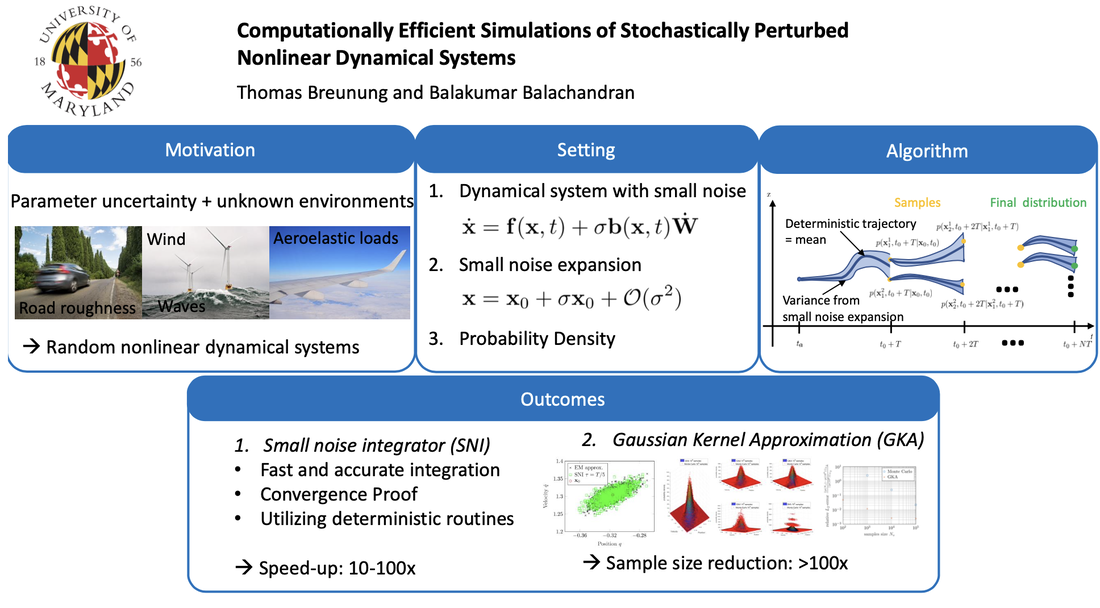
Thomas Breunung and Balakumar Balachandran, Computationally Efficient Simulations of Stochastically Perturbed Nonlinear Dynamical Systems, J. Comput. Nonlinear Dynam. Sep 2022, 17(9): 091008, https://doi.org/10.1115/1.4054932

Dynamical system models can be used to describe natural and engineering systems that evolve in time. Furthermore, most natural processes are inherently nonlinear and face uncertainties stemming from, for example, parameter variations or unknown environmental conditions. Models used to describe such systems can be grouped under stochastic, nonlinear dynamical systems. Here, the authors build on numerical integration routines meant for deterministic systems and present an algorithm to compute responses of stochastic nonlinear systems. With this approach, the well-developed deterministic tools can be used in stochastic system simulations. The algorithm’s performance is demonstrated by using numerical examples, including a system with two-hundred dimensions. This algorithm can be used to compute sample paths of stochastic dynamical systems about two orders of magnitude faster compared to established numerical stochastic integration routines. In addition, a deduced Gaussian kernel enables computations of the time-varying probability density function. With this approach, one can reduce the sample size significantly and thus enable computational investigations of higher dimensional systems that are infeasible with currently available methods. The algorithm discussed here can be used as a basis for computationally efficient investigations into stochastic dynamical systems over long time spans.

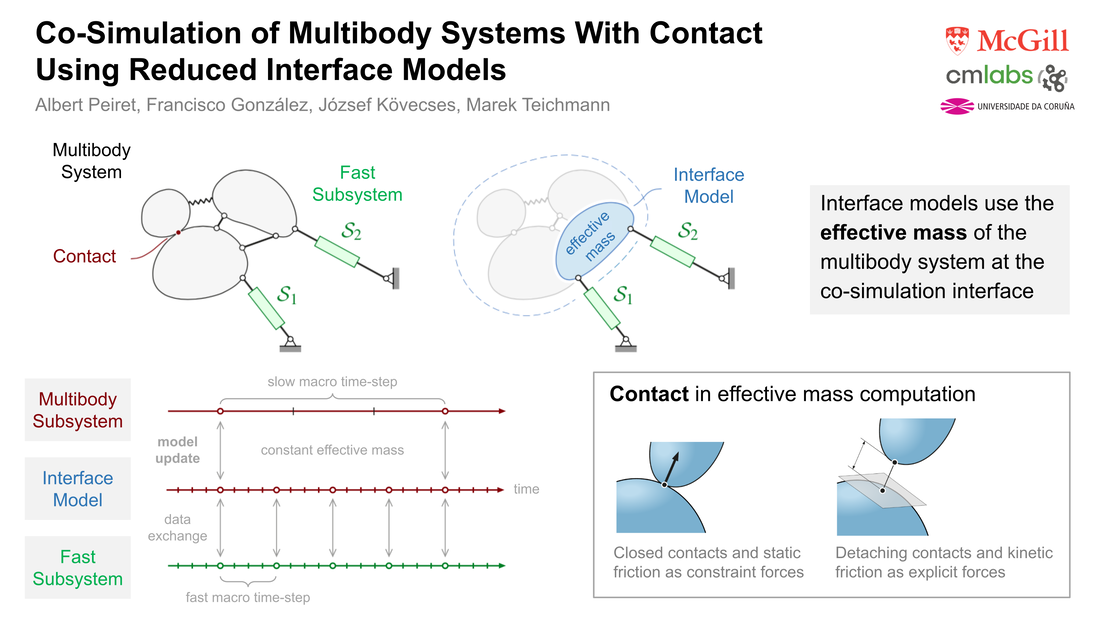
Albert Peiret, Francisco González, József Kövecses​, and Marek Teichmann (February 24, 2020). "Co-Simulation of Multibody Systems With Contact Using Reduced Interface Models." ASME. J. Comput. Nonlinear Dynam. April 2020; 15(4): 041001. https://doi.org/10.1115/1.4046052

Co-simulation techniques enable the coupling of models of physically diverse subsystems in an efficient and modular way. Communication between subsystems takes place at discrete time points and is limited to a given set of coupling variables, while the internal details of the subsystems remain undisclosed, and are generally not accessible to the rest of the simulation environment. This can lead to the instability in non-iterative co-simulation that is commonly used  in real-time applications. The stability of the simulation in these cases can be enhanced using reduced, effective models of one or more subsystems. These reduced models provide physically  meaningful information to the other subsystems between communication points.  This work describes such interface models and their application in co-simulation for nonsmooth mechanical systems subjected to unilateral contact and friction. The performance of the proposed approach is shown in some challenging examples of non-iterative, multirate co-simulation interfacing mechanical and hydraulic subsystems. The use of an interface model improves stability and allows for larger integration step-sizes, thus resulting in more efficient simulation. 

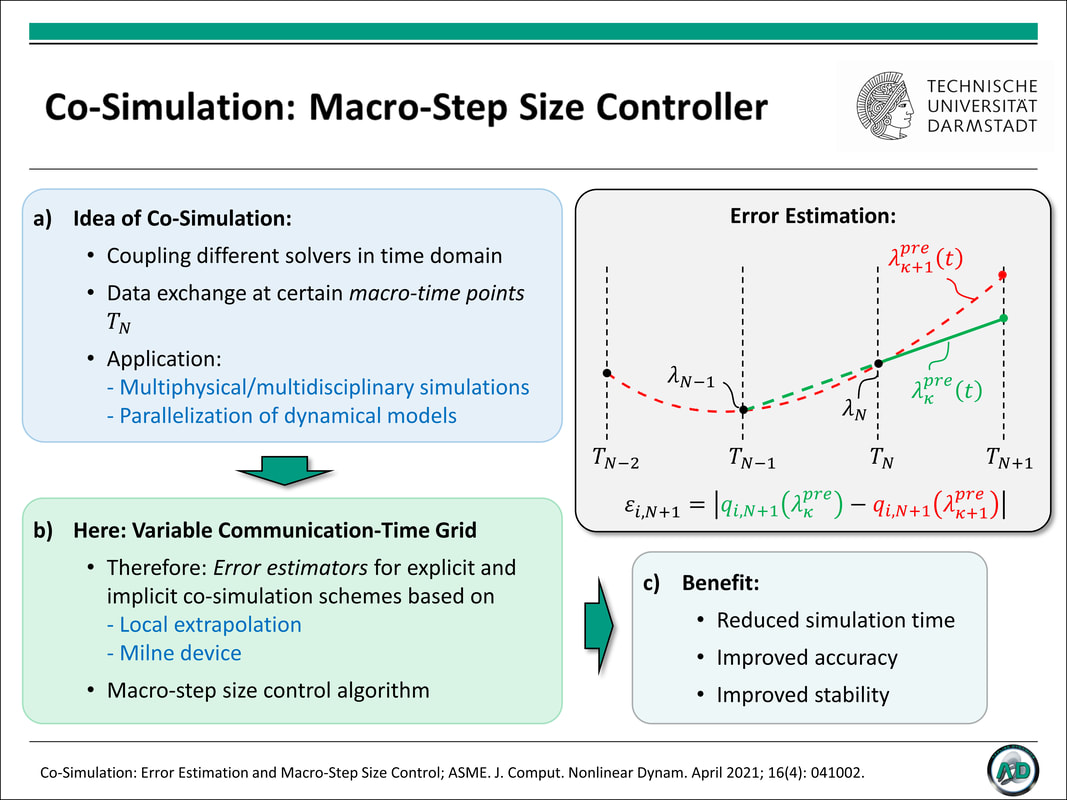
T. Meyer, J. Kraft,  and B. Schweizer (February 24, 2021). "Co-Simulation: Error Estimation and Macro-Step Size Control." ASME. J. Comput. Nonlinear Dynam. April 2021; 16(4): 041002. https://doi.org/10.1115/1.4048944

Co-simulation techniques are commonly used to analyze multidisciplinary and multiphysical systems as well as to parallelize dynamical simulation models. Therefore, the overall system is decomposed into a certain number of subsystems. To define the coupling between the subsystems, coupling equations and appropriate coupling variables have to be specified. Moreover, a communication-time grid has to be introduced by defining macro-time points . The subsystems are integrated independently between the communication-time points; coupling variables are only exchanged at the macro-time points. The crucial point in connection with equidistant communication-time grids, which are frequently used in practical applications, is the appropriate choice of the macro-step size. Efficiency and accuracy of a co-simulation may, however, considerably be increased by using a variable communication-time grid. Therefore, an error estimator for controlling the macro-step size is required. Here, different error estimators for explicit and implicit co-simulation schemes are derived and incorporated into the macro-step size control algorithm. Numerical studies clearly demonstrate that the main problems associated with equidistant macro-grids – namely a trade-off between stability/accuracy and efficiency – disappear, if variable communication-time grids are used. The manuscript focuses on mechanical co-simulation models. The basic results may, however, also be applied to arbitrary, non-mechanical co-simulation models.